van Der Waal’s Equation
Definition
In order to modify the two conflicting
postulates of the kinetic molecular theory regarding volumes and intermolecular
attraction of gas molecules, a Dutch scientist Johannes Diderik (J. D.) van der
Waal (1873) gave mathematical solution by the correction in molecular volume
and intermolecular forces. This is known as van der Waal Equation.
It is the modification
of the ideal gas law to take into account molecular size and molecular interaction
forces.
OR
The equation is
considered as the updated version of the ideal gas equation that states
that there are some point masses present in gases that undergo perfectly
elastic collisions. However, the ideal gas law is incapable of explaining the
behaviour of real gases. Due to this reason, the van der Waal’s equation was
derived to define the physical state of a real gas.
Van der Waals introduced the size
effect and the intermolecular attraction effect of the real gases. These two
effects or factors in the Van der Waals equation are discussed under volume
correction and pressure correction
of the ideal gas equation and are arising because he considered the size and
intermolecular attraction among the gas molecules.
The constants ‘a’ and ‘b’ have positive
values and are characteristic of the individual gas. The van der Waals equation
of state approaches the ideal gas law PV=nRT as the values of these constants
approach zero. The constant ‘a’ provides a correction for the intermolecular
forces. Constant ‘b’ is a correction for finite molecular size and its value is
the volume of one mole of the atoms or molecules.
Kinetic theory of ideal gases assumes the gaseous particles as –
(i) Point masses
without any volume,
(ii) Independent having
no interactions (no forces of attraction or repulsion) and
(iii) Undergo perfectly
elastic collisions.
In practice, van der Waals assumed that, gaseous particles –
(i) Are hard spheres.
(ii) Have definite
volume and hence cannot be compressed beyond a limit.
(iii) Two particles at close range interact and
have an exclusive spherical volume around them i.e. In case of real gases, both
the forces of attraction as well as repulsion operate between gas
molecules.
Note: If the gases obey the kinetic
theory of gases, then they cannot be compressed since the attractions between
the gas molecules is negligible.
Volume Correction in van der
Waal’s Equation
Reason of Subtraction of Excluded
Volume
real gas
molecules are assumed to be a rigid hard sphere with definite volume. As the
particles have a definite volume, the volume available for their movement is
not the entire container volume but less. Therefore, the available volume for
free movement of the gas molecules becomes less than the original molar volume
or volume of the container (Vvessel). Volume in the ideal gas is
hence an overestimation and has to be reduced for real gases.
Proof of Definite Volume of Gas Molecule
(When pressure is applied to a gas, the molecules come closer to
each other. By continuous increase in pressure, a point is reached when
molecules cannot further be compressed since repulsive forces are created. This
indicates that gas molecules have definite volume. Although this is very small
but not negligible).
Volume Correction by Subtracting Excluded Volume from Volume of
vessel
The volume of a gas is the free space
in the container in which molecules move about. Volume V of an ideal gas is the
same as the volume of the container. The dot molecules of ideal gas have
zero-volume and the entire space in the container is available for their
movement.
The volume available for the gas molecules is less than the volume
of the container, V.
Keeping in view the definite volume of gas molecules, van der Waal caluclated the actual volume (available volume) of a gas by subtracting excluded volume of
‘n’ moles of gas, ‘nb’ from the volume of the container (Vvessel). The
volume of a real gas is, therefore, ideal volume minus the volume occupied by
gas molecules.
Available volume = V = Vvessel – b (for
1 one molecule)
V = Vvessel – nb (for
n mole )………………………… (i)
Where
V = free volume/actual volume (molar volume of ideal gas)
Vvessel = Volume of the vessel in which gas molecules
are present
‘n’ = number of moles
‘b’ = volume correction factor/excluded volume/theoretical volume/Incompressible Volume of gas molecules per mole in highly compressed
gaseous state. It is a constant and characteristic of gas, depends upon size of molecule.
The ideal gas equation can be written after correcting for this
as:
P(V– nb ) = nRT
Excluded volume (‘b’) is not equal to
actual volume of gas molecule. In fact, it is 4 times of actual volume of gas molecule.
‘b’ = 4Vmolecule
Where, Vmolecule is actual volume of one mole of a gas.
OR
‘b’ = 4NA x 4/3 𝜋r3
Where
NA = Avogadro’s number
‘r’ = radius of molecule
For a given gas, the numerical vale of ‘a’ is greater than that of
‘b’.
Pressure correction in van der
Waal’s Equation
The pressure of
the gas is developed due to the wall collision of the gas molecules. We know that the intermolecular attraction comes into play when the
molecules are brought close together by squeezing the gas. due to intermolecular attraction, the colliding molecules will
experience an inward pull. Therefore, the pressures exerted by the molecules in
real gases will be less than the ideal gases. The ideal gases
have no intermolecular attraction. van der
Waal also corrected the pressure produced by the molecules of the real gases. The higher the
intermolecular attraction in the gas molecules greater is the magnitude of the
pressure correction term. Therefore, the pressure correction term depends on
the frequency of molecular collisions.
(Gaseous particles do interact. For inside
particles, the interactions cancel each other. But, the particles on the
surface and near the walls of the container do not have particles above the
surface and on the walls. So, there will be net interactions or pulling of the
bulk molecules towards the bulk that is away from the walls and surface. The
molecules experiencing a net interaction away from the walls will hit the walls
with less force and pressure. Hence, in real gases, the particles exhibit lower
pressure than shown by ideal gases).
A molecule (A) in the interior of a gas
is completely surrounded and attracted by the other gas molecules (B)
on all sides. These attractive forces cancel out (The resultant attractive force on the molecule A due to all the
surrounding B molecules is Zero). However, a molecule (A) about to strike the wall
of the vessel is attracted by molecules on one side only, hence it experiences a
net inward pull due to the attractive forces of B
molecules. Therefore, it strikes the wall with reduced velocity.
The pressure of the real gas is less
than the expected pressure due to attractions between the molecules. These
attractions slow down the motion of gas molecules and result in:
i) reduction of frequency of collisions
over the walls and
ii) reduction in the force with which the
molecules strike the walls.
It means
that pressure produced on the wall would be little bit lesser than pressure of
an ideal gas molecule. Therefore, if observed pressure is simply
indicated by P, ideal pressure Pi and less pressure PL,
then equation will be
Pobserved/real or P = Pideal – Pless (∴ Pideal > Preal)
P = Pi – PL
Pideal = Preal +
Pless
Pi = P + PL ------------------------- (ii)
However, the reduction in pressure
depends upon the number of particles (A and B) per unit
volume i.e. is proportional to the square of molar concentration; n/V (one
factor for reduction in frequency of collisions and the second factor for
reduction in strength of their impulses on the walls).
[The average pressure exerted by the
molecules decreased by PL, which is proportional to the square of
the density of gas molecules. Therefore, PL a 1/V2, since density a 1/V; ∴ PL = a/V2]
PL is pressure-correcting
term for real gases, which is the pressure drop due to backward pull of striking
molecules.
Where ‘a’ is a proportionality
constant called van der Waals constant of attraction and is characteristic
of a gas. Higher values of ‘a’ indicate greater attraction between gas
molecules. The easily compressible gases like ammonia, HCl possess higher ‘a’
values. Greater the value of ‘a’ for a gas easier is the liquefaction.
Insert the value of PL in equation (ii)
This is van der Waal’s equation. Here ‘a’ and ‘b’ are van der
Waal’s constants and contain positive values. The constants are the
characteristic of the individual gas. When gas is ideal or that it
behaves ideally then both the constant will be zero. Generally, ‘a’ constant
help in the correction of the intermolecular forces while the ‘b’ constant
helps in making adjustments for the volume occupied by the gas particles.
van der
Waal’s Constant for some Common Real gases
Units for van der Waal’s Constants ‘a’ and ‘b’
From the pressure correction
expression, the value of ‘a’ is calculated. If the pressure is expressed in
atmospheres and volume in liters,
Since P =an2/V2, hence a = PV2/n
But substituting the units of P (atm), V (dm3) and n
(mol), we get
Unit of a = atm dm6 mol−2
Since ‘nb’ is
excluded volume for n moles of gas, ‘b’ is expressed b is expressed in litre
mol–1 units if volume is taken in litres,
b = volume/n =
liter/mol or litre mol–1
Unit of b = dm3/mol, since ‘b’ represent the volume per
mol of gas.
SI units of a and b
If pressure and volume are taken in SI
units, we have
b = volume/n = volume
mol–1 = m3 mol−1
The dimensions of
van der Waal’s constant
Dimensions
of a = [M L5 T−2 mol−2]
Dimensions of b = [L3 mol−1]










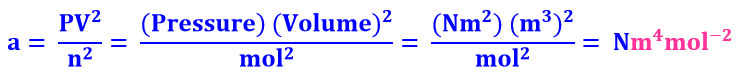



No comments:
Post a Comment